++ 50 ++ α β γ are zeroes of cubic polynomial x3 – 12x2 44x c. if α β γ are in ap find the value of c 264450

SOLUTION 43 36 α, β, γ are zeroes of polynomial x3 px2 qx 2 such that αβ 1 = 0 Find the p (x) = x³ 12x² 44x c Since, α, β and γ are the roots of p (x), α β γ = 12 (i) αβ βγ γα = 44 (ii) αβγ = c (iii) Given that, α, β and γ are in AP, β α = γ α or, 2α = β γ (iv) Now, (i) × 2 ⇒ 2α 2 (β γ) = 24 ⇒ (β γ) 2 (β γ) = 24 ⇒ 3 (β γ) = 24 ⇒ β γ If α and β are the zeros of the polynomial f(x) = x^2 px q, then a polynomial having 1/α and 1/β and is its zeros is asked Apr 24 in Polynomials by Madhuwant (381k points) polynomials;

Cbseworld Weebly Com Uploads 2 8 1 5 Maths 9 Pdf

α β γ are zeroes of cubic polynomial x3 – 12x2 44x c. if α β γ are in ap find the value of c

α β γ are zeroes of cubic polynomial x3 – 12x2 44x c. if α β γ are in ap find the value of c- Solve this â ‹Q16 If α , β , γ are the roots of the equation x3 px2 qx r = 0 , then (â ‹α â ‹β) (â ‹â ‹β â ‹γ)(γ â ‹α) = Math Linear Programming Also we know that if the roots of equation x3 12x2 39x 28 0 are in ap then common difference will be Mathematics TopperLearningcom ez99p5ll Add it here!Polynomials, Class 10 Chapter 2 Extra Question from Rd Sharma for board exam α, β, γ are zeroes of the polynomial x3 px2 qx 2 such that αβ 1 = 0 Fi

If Alpha Beta And Gama Are The Zeros Of The Polynomial Ax3 Bx2 Cx D Find The Value Of 1 Alpha 2 1 Beta 2 1 Gama2 Mathematics Topperlearning Com Ykycwpyy

# QUESTION TEXT If α and β are the zeroes of the quadratic polynomial f(x)=Kx²4x4 such that α²β²=24,find K# FORMULA USED HERE Students can solve NCERT Class 10 Maths Polynomials MCQs with Answers to know their preparation level 1 If one zero of the quadratic polynomial x² 3x k is 2, then the value of k is 2 Given that two of the zeroes of the cubic polynomial axEg 2³2²(8×2)12 = 0

 If α and β are the zeroes of the polynomial x^2 4x 3, find the polynomial whose zeroes are 1α/β and 1α/β asked in Mathematics by damini ( 15 points) polynomial If α and β be the zeroes of the polynomial x^2 10x 30, then find the quadratic polynomial whose zeroes are α 2β and 2α β asked in Polynomials by Anika01 ( 571k points) polynomials41 34 α, β, γ are zeroes of cubic polynomial x3 – 12x2 44x c If α, β, γ are in AP, find the value of c SOLUTION 42 35 α, β, γ are zeroes of cubic polynomial x3 – 2x2 qx – r If α β = 0 then 2q is equivalent to?

 Ex 24, 3 If the zeroes of the polynomial x3 – 3x2 x 1 are a – b, a, a b, find a and b For a cubic Polynomial p(x) = ax3 bx2 cx d With zeroes α, 𝛽 and γ We have 𝛂 𝛽 𝛄 = (−𝒃)/𝒂 𝛂"𝛽" 𝛽𝛄 𝛄𝛂 = 𝒄/𝒂 𝛂"𝛽" 𝛄= (−𝒅)/𝒂 Now, p(x) = x3 − 3x2 x 1 Comparing with p(xα,β and γ are zeros of cubic polynomial and are in AP So, Let β = a ;If the zeroes of the cubic polynomial x3 – 6x2 3x 10 are of the form a,a b and a 2b for some real numbers a and b, find the values of a and b as well as the zeroes of the given polynomial asked in Class X Maths by priya12 ( 12,1 points)

Www Cbseguess Com Uploads Papers th mathematics10th Pdf

Alpha Beta And Gamma Are Zeroes Of Cubic Polynomial X Cube 12 X Square 44x C Maths Polynomials Meritnation Com

 If α and β are the zeroes of the polynomial f(x) = x 2 px q, form a polynomial whose zeroes are (α β) 2 and (α β) 2 polynomials;Class10 If α and β are the zeros of the quadratic polynomial f(x) = x^2 − 1, find a quadratic polynomial whose zeroes are 2α/β and 2β/αQ9 If α and β are zeros of p(x) = x2 x1, then find 1/α 1/β?

Zeros Of The Polynomial Questions And Answers Topperlearning

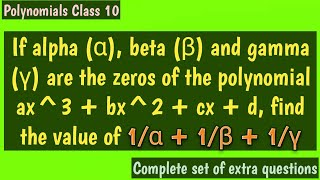
If Alpha Beta And Gamma Are The Zeros Of The Polynomial Ax 3 Bx 2 Cx D Find The Value Of Youtube

• Relation between the zeroes and coefficients of a polynomial If α and β are the bc –zeroes of a quadratic polynomial ax2 bx c, then α β , αβ aa • If α, β and γ are the zeroes of a cubic polynomial ax3 bx2 cx d, then b c–d –αβγ , α β β γ γ α and α β γ a aa • The division algorithm statesQ11 If one of the zeros of the cubic polynomial x3 ax2 bx c is 1, then what will be the product of the other two zeros?P(x) = x^3 ax^2 bx c Since 1 is a zero of P(x) P(1) = 0 Or 1 a b c = 0 Or abc = 1 P(x) ={ x^2 (ab) c (ba1)}*(x1) Product of other two zeros is

Most Important Questions For Cbse Class 10th

Class X Holidays Homework Session 19 Pdf Chlorine Chemical Reactions

 If α and β are the zeros of the quadratic polynomial f(x) = x^2 – 5x 4, find the value of 1/α 1/β – 2αβ asked in Polynomials by ShasiRaj ( 625k points) polynomialsFactor theorem and comparing coefficients write the polynomial in the form ax³ bx² cx d = 0 Guess the first factor from the factors in the last term and extract the first solution from this, plug them into a formula until you get one which = 0 ;Just as a quadratic polynomial does not always have real zeroes, a cubic polynomial may also not have all its zeroes as real But there is a crucial difference A cubic polynomial will always have at least one real zero Thus, the following cases are possible for the zeroes of a cubic polynomial All three zeroes might be real and distinct

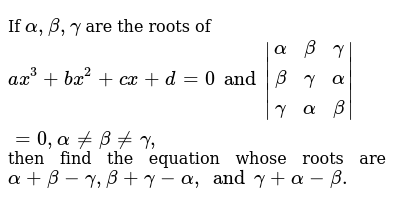
If Alpha Beta Gamma Are The Roots Of Ax 3 Bx 2 Cx D 0 And Alpha Beta Gamma Beta Gamma Alpha Gamma Alpha Beta 0 Alpha Beta Gamma Then Find The Equation Whose Roots Are Alpha Beta Gamma Beta Gamma Alpha And Gamma Alpha Beta

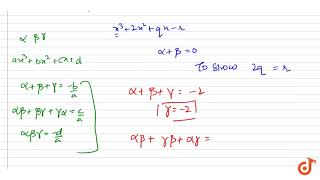
If Alpha Beta Gamma Are Zeroes Of Polynomial X 3 2x 2 Qx R 0 Alpha Beta 0 Then Show 2q R Youtube

Zeroes of ;Avail 25% off on study pack Avail OfferHello guys Zeroes of a polynomial means its roots So let α,β,γ be the roots of a cubic polynomial We can express the polynomial in two forms 1From the roots we can write a polynomial as ( x α)(x β)(x γ )=0 2From knowledge of relation between

2

If Alpha Beta Gamma Are Zeros Of Cubic Polynomial Ax Bx Cx D Write Down The Relation Between Brainly In

1234567891011Next